Discrepancy in Bi-207 gamma emission probabilities

Physics, Transport and Magnetic Fields
1

Dear FLUKA experts,

I am currently working on radionuclide simulation and analyzing emission spectra generated by FLUKA, with the goal of comparing them to those obtained using another Monte Carlo code (PENHAN, based on the PENELOPE code) for the same radionuclides.

In general, I have found good agreement between the two codes for various radionuclides, with some differences explained by different approaches in each code. However, when analyzing the photon spectrum of Bi-207, I noticed a discrepancy I cannot explain.

To provide context on how I performed the calculations in both codes:

  • In FLUKA, I simulated a point source of Bi-207 placed at the center of a void sphere of 1-micron-radius, whose surface acts as a detector of the emission spectra of the source. To account for this detector, I used the USRDBX card (without specifying the detector area, so I understand FLUKA assumes 1 cm^2 area). This gave me the differential fluence dN/dE in units of 1/(GeV*cm^2). If I am interpreting this correctly, multiplying these dN/dE values by the bin width gives the number of particles in each energy bin and, therefore, the energy distribution of particles reaching the detector.
  • In PENHAN, I performed a simulation with an equivalent setup, and with a detector that gave me the probability density (in 1/GeV) with the same energy grid. Since in FLUKA we are assuming a detector area of 1 cm^2, the results obtained with PENHAN should be equivalent to those obtained by FLUKA, though with a different normalization factor which is applied in PENHAN. So while the absolute values differ due to this normalization factor, the shape and relative intensities should still be comparable. In fact, this approach yielded consistent results for other radionuclides.

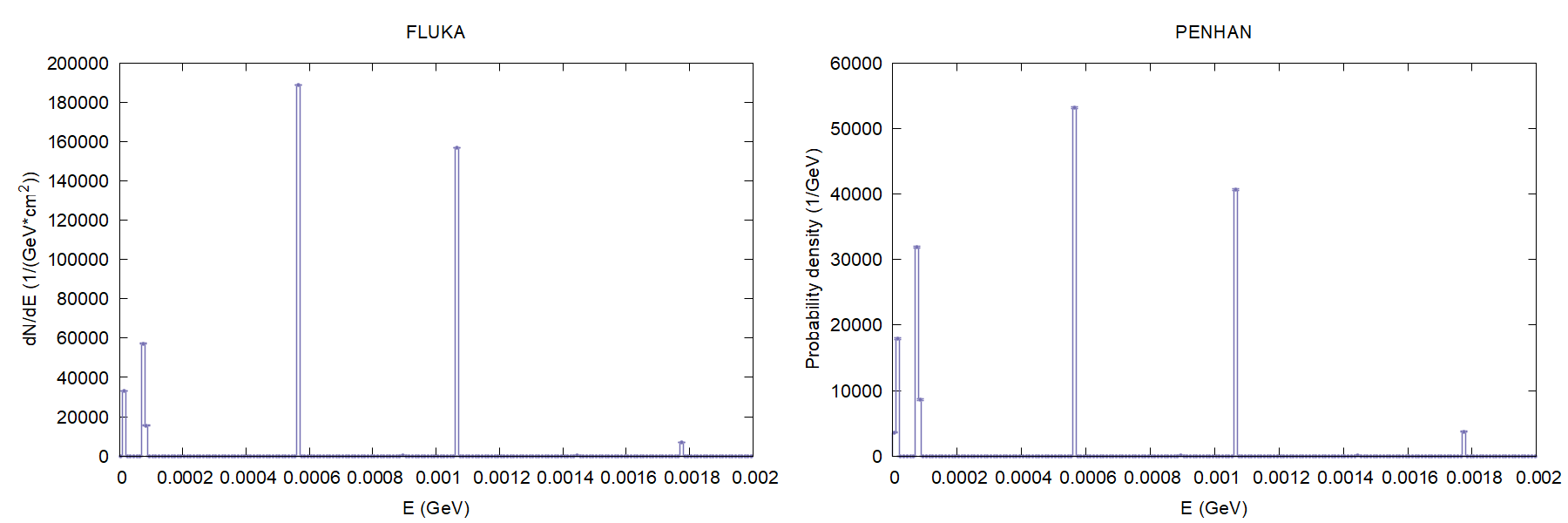
For Bi-207 photon spectra, the graphs obtained were the following:

spc_bi_207
spc_bi_2071800×600 14.3 KB

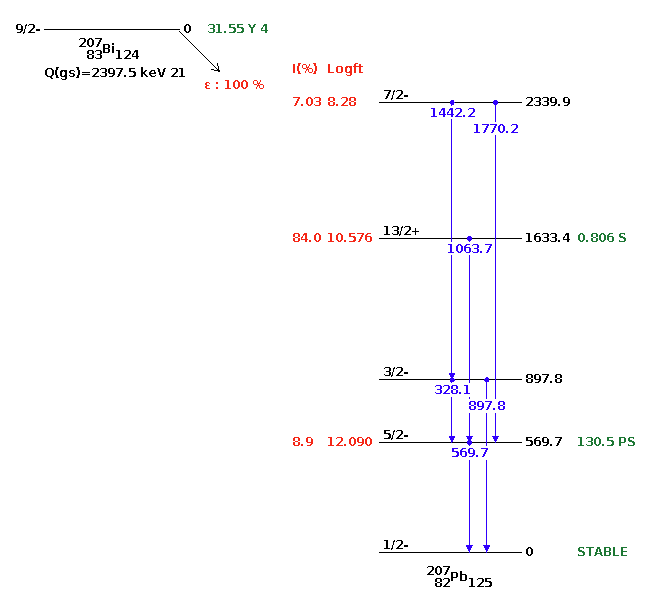
We observe that the relative intensities of the gamma lines at 569.698 keV and 1063.656 keV (that result from the nuclear de-excitation of the daughter nuclide Pb-207) to the other lines are higher in FLUKA compared to PENHAN.

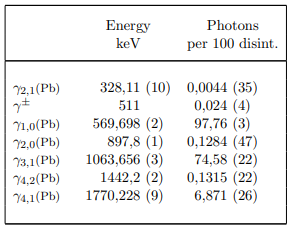
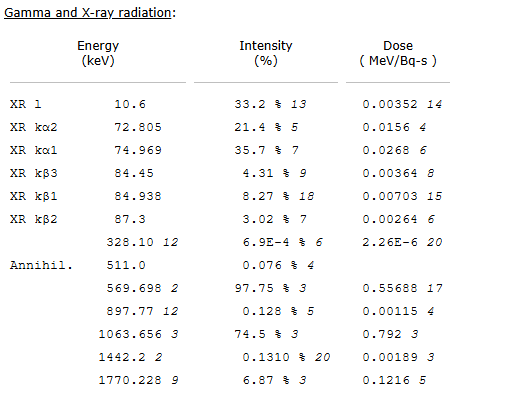
I have checked the decay data used by both codes. PENHAN relies on the LNHB database (Nuclear Data – Table – Laboratoire National Henri Becquerel), while FLUKA (as far as I understand) uses NuDat database (NuDat 3). The gamma energies and intensities listed in both databases are very similar, as shown in the figures below:

LNHB database:

bi_207_LNHB
bi_207_LNHB292×226 9.95 KB

NuDat databse:

bi_207_NuDat
bi_207_NuDat531×401 6.29 KB

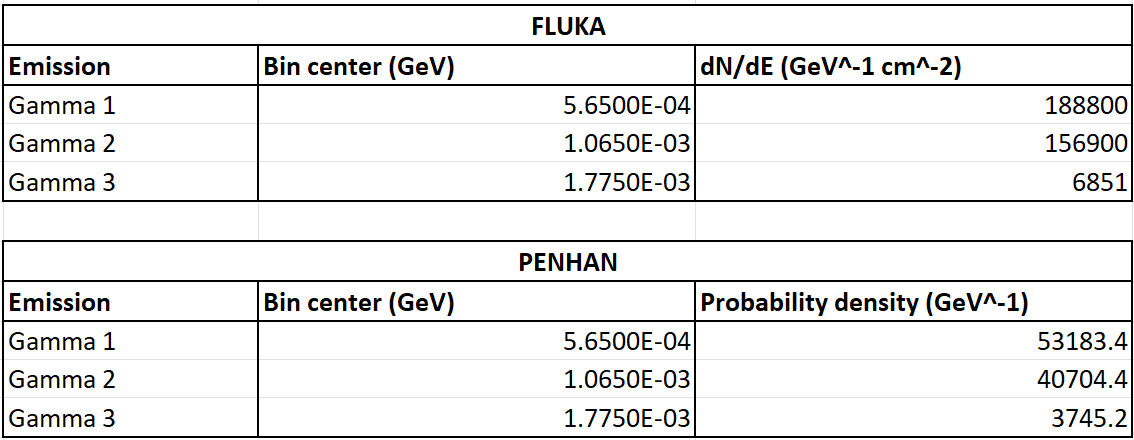
Additionally, we have collected the numerical values obtained by both FLUKA and PENHAN in the table below. For FLUKA, the values were extracted from the _tab.lis file corresponding to the photon detector.

bi_207_fluka_and_penhan
bi_207_fluka_and_penhan1134×440 13.3 KB

With these values, we calculated the relative intensities of gamma1 and gamma2 with respect to gamma3 (simply by dividing) for the four sources: FLUKA results, PENHAN results, LNHB data, and NuDat data. The results are as follows:

bi_207_relative_intensities
bi_207_relative_intensities1414×125 6.71 KB

We observe that FLUKA yields higher values than both the databases and PENHAN. Based on this, it seems that FLUKA may be overestimating the 569.698 keV and 1063.656 keV photon intensities for Bi-207.

Is there anything I might be missing in how FLUKA handles this radionuclide decay or in how I have set up the simulation?

For reference, I attached the input file used in FLUKA:
bi_207.inp (2.0 KB)

Thank you very much for your help.

Best regards,

Lidia

3

Dear Lidia,

I confirm that I can see the same as you, both by running my own independent input and by running yours. After looking inside the database, I also confirm everything is in order, and conforming to the NuDat decay products that you reminded in your post. Note that for Bi-207, we are missing the beta+ spectrum representing approx 0.01% of the decays from Bi-207, but that’s not related to the present question.

I think the issue comes from the fact the NuDat decay products list encompasses all products down to the ground state of Pb-207, whereas FLUKA processes decays up to the next GS/Isomer.

In the present case of Bi-207 decaying EC/B+ to Pb-207, the branchings are as follows

  • 93% ECm (EC to the isomer of the daughter)
  • 6.988% EC (to the GS)
  • 0.012% B+ (to the GS)

So, the isomer is reached in 93% of the case. If you go to the isomer, FLUKA still emits the decay radiation from NuDat indiscriminately. In addition, you would have a subsequent de-excitation of the isomer down to the ground state, which leads to an increase in those specific 2 gammas : 565 keV (added 91% on top of 97.9% so 93% increase) and 1065 keV (added 82.3% on top of 74.6 so 110% increase). (edits in italics)

This is something we are looking to correct in the wider context of the overhaul of the isomer treatment in FLUKA, however we can’t bring a quick fix easily, as this requires a change in the decay logic of the code.

Cheers
Philippe

4

Dear Philippe,

Thank you very much for your prompt response.

After reviewing the information you provided on the decay of Bi-207, I have a few follow-up questions:

  • I’m not sure which Pb-207 isomer you are referring to. I’ve retrieved the decay schema from the NuDat database (available at: Decay Scheme), and included it below for reference:

bi_207_decay_schema
bi_207_decay_schema665×593 3.42 KB

Could you confirm whether the isomer you’re referring to is the state at 1633.4 keV with a lifetime of 0.806 s, or if it’s a different one?

  • Regarding the branching ratios you mentioned, I couldn’t find those values in NuDat database. Could you kindly provide the source or reference where they are reported? Looking at the decay schema in the above image, there is no transition from Bi-207 directly to the ground state. Additionally, I couldn’t find a transition to any level with a branching ratio of 93% as you mentioned.

  • Finally, regarding the ~93% increase you noted for the 565 keV and 1065 keV gammas, I checked it with the numbers I provided in Figure ‘bi_207_relative_intensities.png’ of the first post for FLUKA and NuDat results. For Gamma1/Gamma3 ratio, I calculated 27.55802073/14.22853= 1.9368, which indeed corresponds to a ~93% increase in FLUKA relative to NuDat. However, for Gamma2/Gamma3 ratio, I obtained 22.90176617/10.84425= 2.1118, indicating a higher increase (~111%).

Could you please give me some clarifications regarding these points?

Thank you again for your support.

Best regards,

Lidia

5

Hi Lidia,

I confirm, the isomer in question is the level at 1633 keV, with a hl of 0.8s.
The branchings i gave you in my first answer are extracted from the internal FLUKA database, and they result from the current treatment of isomers in FLUKA, where decays towards levels at or above the isomer are assumed to end at the isomer, and the ones below, to the ground state (with the listed gammas/electrons as radiation products).

As I said, we are looking to overhaul the logic behind the determination of these branchings, which in this case are inaccurate: the percentage of EC decays passing through the Isomer is 84%, not 93%. This figure is easy to determine in this particular case, but in general can be tricky for more complex level structures. That’s why this percentage is calculated using a general algorithm that will be improved in the future.

In addition, we have the issue of the decay from the isomer to the ground state producing additional decay radiation even though this is already accounted for in the NuDat data, and therefore in the FLUKA code. This is something that will be solved after we transition towards the new isomer treatment.

For this particular case, here is the arithmetic in detail:

Bi-207 has three decay channels

  • 93% EC towards the Isomer
  • 6.988% EC to the GS
  • 0.012% B+ to the GS

In ALL three cases, we produce the decay radiation associated with the decay of Bi-207, which is essentially the NuDat data you cited above. In particular here are the branchings for the 5 gamma rays: (i skip electrons and X-rays)

  • 97.9% @ 570 keV
  • 0.13% @ 898 keV
  • 74.6% @ 1064 keV
  • 0.13% @ 1440 keV
  • 6.87% @ 1770 keV

If FLUKA happens to have sampled a decay to the Isomer (93% of cases) you end up on the Isomer, which then deexcites.
The decay radiation for the isomer is the following: (see NuDat for Pb-207)

  • 97.9% @ 570 keV
  • 88.5% @ 1064 keV

What you score is the sum of all of this, resulting in a

  • (97.9*0.93 + 97.9) / 97.9 = factor 1.93 overestimation for the gamma @ 570 keV
  • (88.5 * 0.93 + 74.6) / 74.6 = factor 2.1 overestimation for the gamma @ 1064 keV

This is the explanation of the numbers you obtain (I have edited my first message to make this clearer). This is somewhat unsatisfactory of course and will work towards a more accurate and robust solution for all nuclides.

Cheers
Philippe