Dear experts,
I would like to know what is the meaning of the statistical error of FLUKA simulation results?
I saw the description of statistical error in the PDF of the course, but the concept of statistical error is still unclear.
How does the statistical error relate to the results of each cycle in the simulation?
How does the statistical error relate to the variance calculated in the course?
Can you give a clear idea of the statistical error?
Dear Junjie,
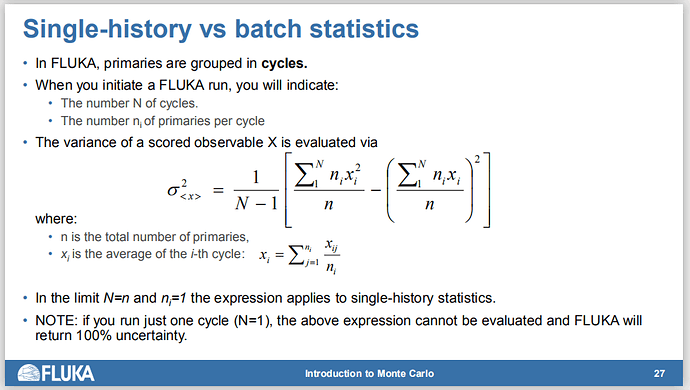
The error estimate provided by FLUKA for an observable is related to the cycle-to-cycle variations of that observable. For example, if the variance of the fluence values (x_{i}) associated to a bin of a USRBIN detector obtained after running 5 (N) cycles is large compared to their mean estimate (\left\langle x \right\rangle), you can expect the relative statistical error to be large. Therefore, the last statement on your slide is true, if only one cycle is run, the variance, hence the statistical error, cannot be determined because the cycle-to-cycle variations are unknown.
The variance estimate is calculated according to the formula shown on your slide. There, the values of the observable of interest obtained after each cycle are x_i. The values n_i are the number of primaries (or primary weights) simulated during each of those cycles. n is the sum of the n_i values, or the total number of primaries (primary weights) in all of the cycles combined.
The values x_i are the cycle-mean estimates of the observable values x_{ij} obtained after simulating only one primary.
The standard deviation estimate, \sigma_{\left\langle x \right\rangle}, interpreted as the error on the observable, is obtained by taking the square root of the variance \sigma^2_{\left\langle x \right\rangle}. The relative error is taken as \sigma_{\left\langle x \right\rangle} / \left\langle x \right\rangle whereby \left\langle x \right\rangle is the mean estimate of the observable obtained when using the combined information of all cycles.